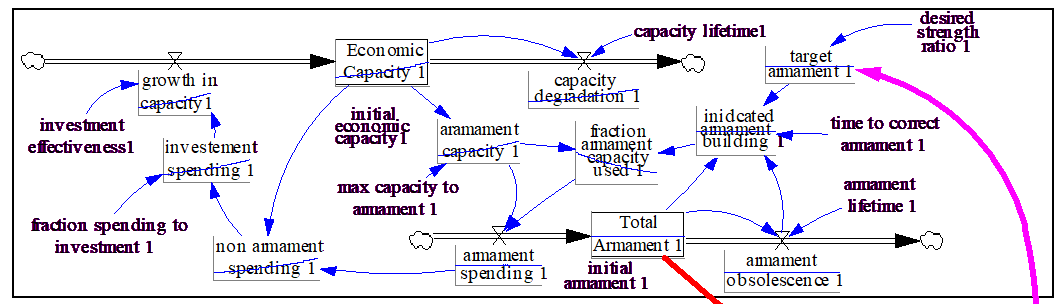
One of the most important features of exponential growth is that there is, seemingly, very little activity for a long period of time, and then an explosion. In this model the explosion is contained because the conserved flow of Adoption into Practitioners also depletes the source of growth, the stock of Non Practitioners. If we break this link in our model, replacing the equation for the level Non Practitioners with a constant:
Non Practitioners = 1E7
and, to make it true exponential growth:
total population = 1E7
We remove the constraint on growth.
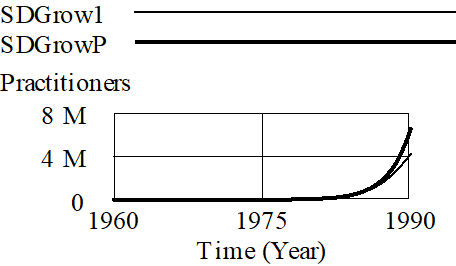
The models diverge after 1989 very dramatically - pure exponential growth makes the diffusion process look like a flat line at 0. Before 1988, however, the two models give very nearly identical results. The graph on the right is a close-up of 1960 to 1990, and there is almost no difference between the two models until near the end of this time.
For this model, the implied growth rate is just
contact rate * adoption fraction = 100 * .005 = .5
or 50%/year. With adoption fraction set at .001 there is only a 10%/year potential growth rate, thus the big difference in takeoff.